According to one source, Barney is in a triangular room ABC. He walks from a point on BC parallel to AC. When he reaches AB, he turns and walks parallel to BC. When he reaches AC, he turns and walks parallel to AB.

 The purpose of this exploration is: (a) To prove that Barney will eventually return to his starting point; (b) To determine how many times will Barney reach a wall before returning to his starting point; and (c)To examine the extensions and interpretations of this exploration.
 We begin by exploring  and discussing   various starting points on line BC, including points exterior to segment BC.

Let us consider two trivial points on BC.
 
Case1. Let Barney begin at vertex C.

If he walks parallel to AC then he will be walking on AC. Thus for this starting point he will be walking around the triangle on the sides of the triangle.

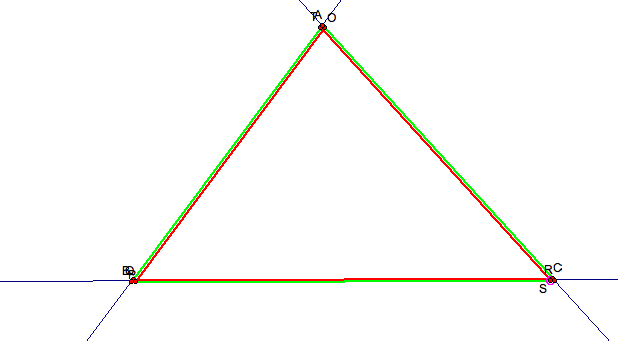
This case the total distance traveled is the perimeter of the triangle, and he will only have to make on revolution to return to the starting point.

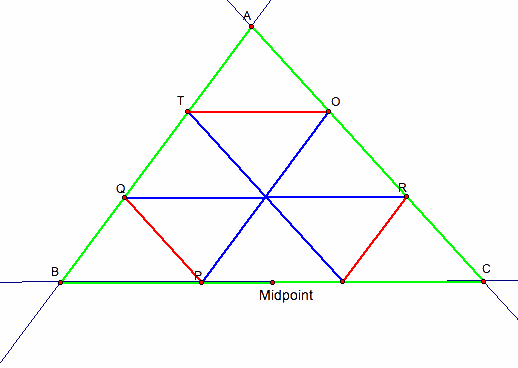
Case 2. Let Barney begin at the midpoint of BC.

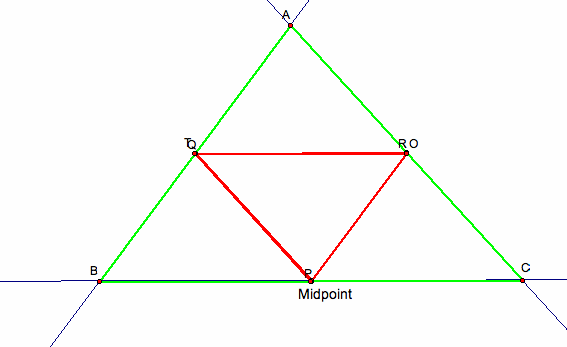
For this point, if we walks parallel to AC, then the mid segment theorem for triangles states that he will hit the mid point of of  AB.Similarly from here he will hit the mid point of AC then Back to the mid point of BC which is his starting point. By the Mid segment he would have traveled half the perimeter of triangle ABC.

 For these two trivial cases, Barney returned to his starting point. We can now conjecture that he will always return to his starting point.
                
Thus far we have (We count his return as hitting the wall, otherwise the number of hits would be 2 for the mid-point and 5 for any other point on segment BC)
 

Starting Point (Equal parts of BC)	1	3	4	5	6	n
number of times to hit the walls	3	6	6	6	6	6
Distance (In terms of Perimeter	1	1	1	1	1	1

Suppose we can divide BC into any number of equal parts as we wish.
We will use the side-splitting Theorem in this investigation:
 Side Splitting Theorem:
 A line parallel to a side of a triangle that intersects the other two sides in distinct points splits these sides into proportional segments.

Using the side splitting Theorem, and the set of similar triangles formed, we have:
 For 3 parts we have 1/3(a + b+ c) +2/3(a+ b+ c) = a+ b + c. Note that in the figure below, the three red segment plus the three blue segment is the perimeter of the triangle.

 

Similarly, for four parts we have 1/4 (a +b +c) + 3/4(a+ b+ c) = a+b+c, and for n parts we have 1/n(a + b +c) + (n-1)/n (a+b+c) = a+b+c

Therefore for all points on segment BC we will always get similar triangle and we will always get back to the starting point because, given any point. the ratio remains constant for that path. and the side-splitting theorem guarantees we will get back to the starting point.

What if we do not have the side splitting Theorem??

 Then by the definition of Barney's path, we have parallelograms and given any starting point on BC we will get a set of parallelograms. We then use the property of parallelograms to prove congruent triangles.

 

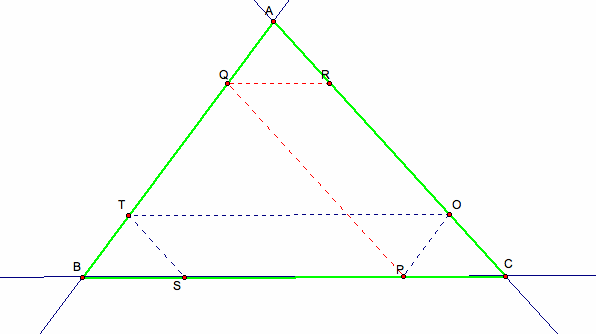
 Let Barney begin at point S on segment BC, goes to point T on segment AB then to point O on AC then stop at point P on BC. We then have two parallelograms.
 Parallelogram BTOP and parallelogram  SCOT. Therefore, since opposite sides of a parallelogram are congruent, we have triangle BTS congruent to triangle POC by the AAS congruence condition.
Now Barney continued up to point Q on on segment AB then to R on segment AC and rested. We note that we have two new parallelograms, PQRC and PQAO. Therefore By the AAS congruence condition triangle  QAR is congruent to triangle POC.
 Thus by transitivity of triangle congruence, BTS is congruent to QAR. Therefore BQRS is a parallelogram. Hence Barney returns to the starting point.
Now this starting point was arbtrary on segment BC, and so Barney will always return to his starting point.


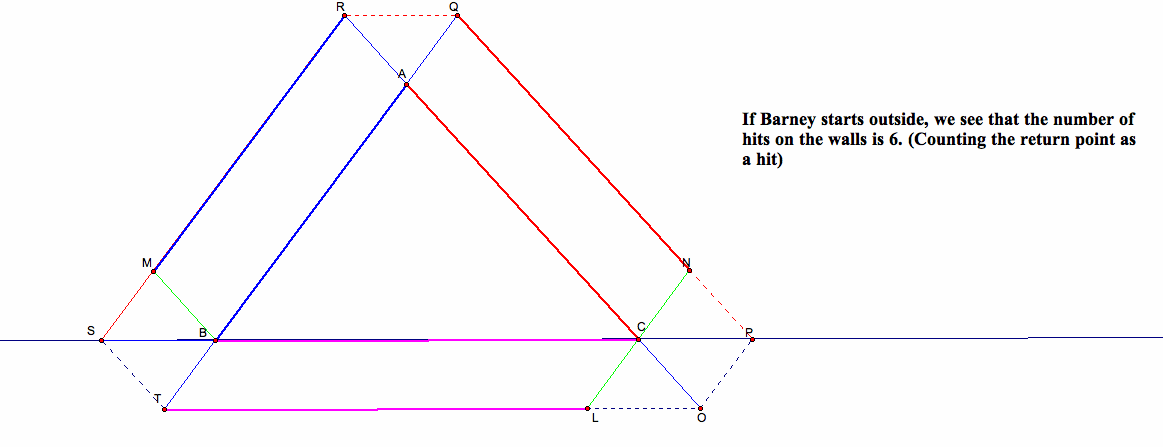
Now suppose barney start from some point outside the triangle?

We get the distance Barney traveledwhere SB is the distance away from the veterx that Barney begins.

Please OPEN GSP file (Page 5) to see the proof

---

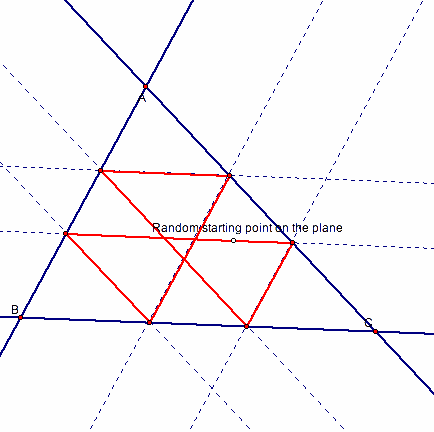
Now we can look at several orher cases with Barney in this triangle or outside the triangle. Suppose Barney begin at some random point on the plane, then we get a similar analysis

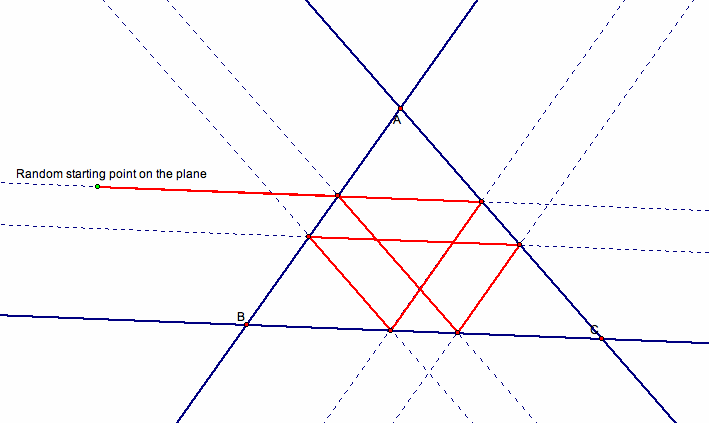
But what if barney was trapped in a pentagon? Octagon? n-gon?

What value of n will Barney not return to the starting point? What if Barney was inside a circle? How would we define the path? What if there were more restrictions to barney's path?

Please OPEN GSP file to continue this investigation

Return